I covered the photocurrent already before, for instance here. 
I pointed out that from the light intensity dependence of the short circuit current, it is impossible for many typical conditions to unambiguously determine the dominant loss mechanism or even the recombination order (1st (often called monomolecular, but not my favourite term;-) or 2nd order of decay).
If, however, you know (or guess) that the recombination order is two, you can use the above mentioned 
The TPV response is approximately a simple exponential decay, which is strikingly different from the power-law form of the TPC. The time constant decreases from ∼1 ms to 18 “μs for Voc increasing from 0.269 to 0.516 V. Again, this is a completely different result from the form of the photocurrent transients, which have a different magnitude of response time, depend much less on the voltage, and the voltage dependence is in the opposite direction.
As you may know, TPV=transient photovoltage, measured at open circuit, whereas TPC=transient photocurrent is determined under short circuit. One point against his argument is: TPV is a small-signal method, in which Voc is changed only by a few percent due to a (sufficiently weak) laser pulse in addition to bias illumination. Therefore, it decays monoexponentially. In contrast, TPC is a large signal. Bob Street points out that TPC at short circuit decays with a power law. Let me add that is similar to the large-signal method at open circuit, namely the time dependent open circuit voltage. The latter also decays with a power law, and not monoexponentially. Thus, no principal difference between recombination at short circuit and open circuit.
Finally, a nice publication concerning these topics is [Dibb 2011]. From the abstract,
We show that it is only safe to infer a linear recombination mechanism from a linear dependence of corrected photocurrent on light intensity under the following special conditions: (i) the photogenerated charge carrier density is much larger than the dark carrier density and (ii) the photogenerated carrier density is proportional to the photogeneration rate.
Indeed, it all depends;-)
And lastly (does this come after finally? ;-) a few links I found over the weeks. From the Coronene Blog, an excellent parody of how to publish high impact papers. An insightful article on mistakes in scientific programming by Zeeya Merali in Nature News. A list of the Top 100 Material Scientists, from Thomson Reuters based on impact factor etc – despite that, interesting;-) What’s wrong with scholarly publishing? How it used to be on petermr’s blog. Then: Is there a point in publishing corrections to articles? It seems not (Scholarly Kitchen)! Infodocket: Google Scholar Citations launched. On Nature Chemistry: The art of abstracts (similar to some others, behind the wall…). You know that I like preprint servers, as they are barrierless: arXiv just turned 20, written by Paul Ginsparg himself for Nature. Then nice pic: how people in science see each other…as always, there is some truth to simplifications;-) Nice article by Ben Coldacre on paywalls for science, Academic publishers run a guarded knowledge economy (Guardian).
That’s it. If you wonder why I have time to write, I have one month “off”: we call it parent time in Germany, it is my part 2 (part 1 was directly after the birth). See you again, still have to finish this other science thing…
Notes on Disordered Matter 
Dear Carsten
Where can I find more information on the large-signal method at open circuit?
Thank you
Regards
Nissy
Dear Nissy, thank you. There is not much in literature for organics, as far as I know. The method is simple enough and described in the literature for inorganics under the name open circuit voltage decay: hold your device at zero current, laser pulse, measure the resulting Voc vs time. One paper for dye cells is [Zaban 2003] and I believe the coauthor Juan Bisquert has also done these experiments on organic solar cells. Regards, Carsten
Thank you Carsten!
Dear doctor Deibel;
I’m so happy to see you and i congratulate you for your research on organic solar cells
Let me introduce my self,my name is khadidja,i’m algerian and i’m student.
I hope that you could answer me.
currently I am preparing my master thesis,i’m working in modeling of organic solar cells using matlab,i have some difficulties in order to calculte the dissociation probability of electron hole pair using matlab,the formula of integration the probability is very long and depends on many variables,like the elctric field wish is introduced in the formula of b ,knowing that the electric field is descritized using finite difference method, do you see? also we have to integrate the probability over all charge-separation
distances (we have infinit integral),could you tell me at wish value we born the integral? could we using the initial separation distance??
please sir ,I need your help.
yours sincerly
Hi khadidja. First off, keep in mind that polaron pair dissociation is not critical in state-of-the-art solar cells: you could first model without that influence. Second, the extension to use a distribution of pair radii comes from the Blom group. While in principle a good idea, no experimental values for the radius, let alone the distribution are known: these are fitting parameters — and more fitting parameters give a better fit. Concerning the length of the formula, it is not that hard without the radius distribution integral, try it without: once working, you can extend it, and you have your first results without distribution as reference values for verification. Nevertheless, I’d start without Braun-Onsager and concentrate on nongeminate recombination. Best, Carsten
Hi Mr.Carsten; thank you very much for your answer,it’s very kind of you.
so I’m inorder to develop code calcul to solve the differential equation system of the Poisson, continuity and drift–diffusion equations by an iterative approach described by Gummel and Scharfetter (i model koster-Blom’s model). could we say that is it the appropriate model to mdel the BHJ organic solar cells?
I have seen your publication (jun 2008), you have introduced a novel prefactor in langevin recombination rate,why?
According to your explanation i understand that i have to calculate dissociation probability without the radius distribution integral, after i do that i.e i do the sum from 0 to rpp (separation length) of P(rpp,T,F) product f(x,rpp) inorder to do the comparision?
You said that “I’d start without Braun-Onsager and concentrate on nongeminate recombination.” did you mean that geminate recombination play a minor role? and the net generation rate is as following: U=G-R ? please sir if you have a buplication speaking about that ,could you let me know??
I apologize for the inconvenience,olso excuse me for my poor english ;)
with my thaks advance
Hi,
the Koster model is fine, , only the application of the Braun-Onsager part was criticised for the use of polaron pair (geminate) lifetimes on the microsecond instead of the experimentally measured nanosecond scale. Alternatively, you can have a look at [Deibel 2009] and references therein.
Geminate recombination and why it is almost field independent in the fourth I-V quadrant of most solar cells: see previous blogpost on geminate recombination and [Kniepert 2011 ]. Thus, indeed, you can neglect Braun-Onsager for getting started.
Nongeminate recombination and why the Langevin prefactor is reduced, see previous blogpost on nongeminate recombination, or [Deibel 2009] or for an overview my review.
Carsten
concerning my last question i would say: we have not appear the dissociation of polarons in the novel approach !
i have the question if I-V characteristic based in this model (neglect od braun onsager) is in a good agreement with experimental characteristics?
Best.Khadidja
That is what I was saying, yes. C
I apologize for my questions wich seem to you so trivial:
sir i have another question concerning the model:
does this model can represent the real behavior of organic solar cell,because in this latter we have the disorder,intern morphology (phase separation),the hopping…
I hope that my questions don’t cause incovenience for you
The effective medium approach, donor:acceptor blend as “one new material”, works quite well for many phenomena. Energetic disorder and hopping are well represented by the mobility model (e.g. the Bässler model for a start). C
many thanks
Hi Mr.Carstern.How are you?
I have a problem when calculating the initiale value of elctrostatic potential in BHJ OPV!! in conventional solar cell we have 2 regions and the doping profil,so we can initialize electrical potential using condition of charge neutrality. in case of OPV we have intrinsic blended D/A heterojunction.can you help me to get the formula of potential inorder to use it as initial value?
Best.
Hi Khadidja, I do not see that there is a problem: the organic BHJ as an effective medium is just an ambipolar device, otherwise with the same equations being valid. Also, as sorry as I am, but the implementation is your job;-) If you have questions concerning the physics, come back here. Carsten
Dear Dr. Deibel,
I enjoy reading your papers. In PRB 82, 115306 (2010), you modelled the S-shape curve. In that what were your boundary conditions? Assuming an ohmic condition and in equilibrium one can take n and p at the boundaries from the eqn 8 & 9 in your paper. Then how could you include the surface recombination rate into the equation? That part I could not understand from your paper. Thanks in advance.
Hi Suman, as boundary conditions for a system with finite surface recombination, equations (8) and (9) cannot be used since they describe a thermionic emission contact in thermal equilibrium. Hence equation (12) (shown only for electrons) has to be used. This approach results in dynamic boundary conditions which have to be adjusted for every calculation step.
Kind regards, Carsten
thanks Carsten,i have done the implementation but i had error in calcul,for this reason i would just ensure what i’m doing.
thank’s a lot.
Hi Carsten :),please I would asking you for grid spacing (mesh size) of MIM stucture,one must choose it smaller than debye length?when I have done calculation I have got a mesh size larger than intrinsic debye length :(!!
Hi Khadidha, I would go for less than one nm for now, irrespective of the potentially higher calculation time. Later on, once your simulation is established, you can try an adaptive grid. Carsten
ok,thank’s.Best
Hi Carsten, If I understood well you are “on vacations” because you being a father again. Am I right? If so, congratulations. Not everything is a bout OPV….:-)
Thanks, Emilio! I was on paternity leave in March and September (not “between” unfortunately;-), so back to work already for 6 weeks or so. You are very right, however, there is much more to life (in the true sense of the word) than OPV ;-) Best, Carsten
jejejeje, indeed!. We are now working on solution processed small molecule solar cells and applying some of your models and techniques to our IV curves as well as applying our CE and TPV. It seems that is very likely that different materials that lead to different morphologies have very much different recombination kinetics. The ones which make crystalline domains seems to be controlled by non-geminative recombination dynamics ( bi-molecular) . Yet, for some of the “amorphous” films we cannot discard the role of geminate recombination.
Let’s see if we can get together at some conference to discuss it. Perhaps you could come over for a conference here if you are not busy early next year.
I agree on the role of morphology. Meeting sounds good. Will you be at MRS Fall this year (I’ll be there), or MRS Spring next year (I’ll be there if the talk is accepted)? And meeting up in Spain always sounds good:-) Best, C
Hi,Carsten,i’m Linda, i asked you a question ten days ago, i didn’t realized that you could give me such a quick response, that’s a surprise, thank you very much! Now i have another question, some publications said they can illustrate the recombination mechanism by transient photocurrent and photovoltage measurement. Although I have read some papers about this, i just cannot clearly understand the principle of this experiment. Does the transient photocurrent and photovoltage measurement can get two kinds of information such as charge transport and recombination? How can i understand this measurement in a simple way?
I also have read one of your papers about the recombination dynamics (Influence of phase segregation on the recombination dynamics in organic bulk heterojunction solar cells), you mentioned that charge carrier decay order is as a function of temperature, i don’t understand the reason for this. Is it necessary to do the measurement at different temperatures? Thank you very much!
Best wishes, Linda
Hi Linda,
transient photovoltage is on an illuminated (with, say, 1 sun) solar cell under steady state conditions. You set the voltage to the open circuit voltage Voc, current=0. An additional laser pulse will change Voc by a small amount dVoc. Make sure that the laser pulse is small enough so that dVoc << Voc (say, 5%, but depends). The excess charge carriers will recombine until the steady state Voc is reached again. As dVoc is small, the decay is exponential, with an effective lifetime tau which is valid for the illumination intensity, here 1 sun. If you do this experiment for different illumination intensities (corresponding to generation rate G), you get a set of effective lifetimes tau(G). Second step: transient photocurrent or charge extraction experiment to determine the carrier concentration n at Voc for the different G, so that you get n(G). Thus, you now have two sets which you can combine, tau(n(G)), thus tau(n). This corresponds to a recombination rate R=n/tau(n), which is usually not a first order process as tau is not a constant with increasing n. If you compare to the recombination rate written in other terms,
, you can find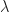 
from your above measurement.
The order of decay
is a function of temperature. A very good starting point to read about this is [Shuttle 2010]. The authors find that at room temperature, $R(n)=k n^{\lambda+1}\propto \mu(n) n^2$, thus 2nd order bimolecular recombination with a concentration dependent mobility responsible for increasing the recombination order above the expected value of two. However, we think the results need to be generalised if you look at lower temperatures, but that is a bit more complicated. For a first glimpse, you could have a look at these slides of ours, Deibel 2011 nanopv presentation, especially slides 15-18.
Regards, Carsten
Thank you very much! Carsten. you are so kind!
Best wishes, Linda
Dear Dr Deibel,
In literature, I find two types of boundary condition (BC) used. Koster et. al. used charge carrier density as BC for ohmic contacts and Barker et al used current boundary condition for non-ohmic contact with barrier height 0.5 eV. From the previous comment, as I get it (I may be wrong), you used current boundary condition for ohmic contact to describe S shape curve. My question is that is it necessary to use current BC to include finite surface recombination velocity? And what is the functional form you used in your paper to calculate Js? best regards, Suman
We used thermionic emission, not ohmic conditions. However, the carrier concentration is not fixed but influenced by the BC for surface recombination.
Thanks a lot! I shall keep reading your paper and disturb you..:)