Recombination of free charge carriers in materials with a low mobility  is often described with the Langevin recombination rate [Langevin 1903 (Ann. Chim. Phys. 28, 433)] (Update 3.12.2008: wrong reference previously, sorry.) Generally, if electron and holes – being potential recombination partners – wish to recombine, the effective recombination rate is proportional to
is often described with the Langevin recombination rate [Langevin 1903 (Ann. Chim. Phys. 28, 433)] (Update 3.12.2008: wrong reference previously, sorry.) Generally, if electron and holes – being potential recombination partners – wish to recombine, the effective recombination rate is proportional to
- the “direct” recombination rate
- finding each other
In high mobility semiconductors, the former is dominant. However, in disordered solids, and particularly disordered organic semiconductors, the low mobility limits the effective recombination rate. The process of finding each other can be described as diffusion limited, which is proportional to the charge carrier mobility when considering the Einstein relation.The idea is as follows (after [Pope & Swenberg 1999]): we assume a negative charge to be fixed, 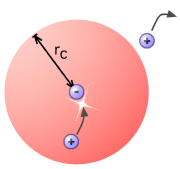 and a positive mobile charge (moving with mobility of electron plus hole), both being attracted by the Coulomb force. The hole can avoid recombination at zero field only if the thermal energy is sufficient to overcome the Coulomb potential. As demarcation line, the Coulomb radius is defined by equating
and a positive mobile charge (moving with mobility of electron plus hole), both being attracted by the Coulomb force. The hole can avoid recombination at zero field only if the thermal energy is sufficient to overcome the Coulomb potential. As demarcation line, the Coulomb radius is defined by equating
as
If we consider now a drift current density for the mobile hole,
bearing in mind that electric field is the spatial derivative of the potential (energy), we get
Now considering that recombination of the two charges takes only place if they find each other, i.e., the hole comes to within the Coulomb radius, we can calculate the hole recombination current. That is the current density flowing into the sphere of radius
around the electron,
with gamma being the Langevin recombination strength
Now, indeed, the recombination is proportional to the charge carrier mobility of electron and hole, i.e., to the two carriers finding each other.
The Langevin recombination strength time electron density times hole density is then the Langevin recombination rate, as used in a typical continuity equation
with generation term G0, a monomolecular recombination term, and the bimolecular Langevin recombination rate.
Bimolecular recombination in disordered plastic solar cells is usually described with the Langevin theory. There seem to be some adjustments to be made, which we’ll discuss another time;-)
(Update 3.2.2009: support by WordPress, excellent! My splendid MarsEditblog editor transfers it, but does not parse it… but that would have been asked too much indeed! No, I am glad it works as is:-)
(Update 18.1.2011: Wapf asks, Carsten answers;-) The thermal energy with is only an estimate as compared to the
for three degrees of freedom. Also, if you are wondering why
, but
: I did not transform
into
;-) Concerning
: consider that a current
. Therefore
“disappears” going from current to continuity equation. Concerning the “appearing”
: the recombination current as calculated by Langevin considers particles which flow into the
-Sphere around the center charge. By definition of Langevin, all charges (here, holes) flowing into the sphere recombine with the center center charge (here, one electron). Going from microscopic consideration to macroscopic equation, this one charge of course has to be translated to a charge density, as this can happen with every electron. (And all of this is of course true for the opposite case, electrons flowing into the “hole sphere”, but this is generalised already in the final rate equation.)

Clear!
Yet again nicely presented!
I have a few questions. Calculating the Langevin recombination strength we assume that the hole is moving in an electric field equal to that generated by two point charges seperated by Coulumb radius? Why? I realise if they don’t get within Coulumb radius then thermal energy is sufficient to escape recombination and if they do get within Coulumb radius then they recombine. Is that so? Could you clarify this point to me, thank you
And in the last formula why is the recombination proportional to langevin recombination strength times electron density n? Why not n^2? I realise it should depend on both carrier densities but why a simple multiplication?
thank you
Hi! Langevin’s theory is very simple but pretty effective. Of course, both electron and hole move, and if they come to within the Coulomb radius, the Coulombic attraction exceeds the thermal energy sufficient for escape. The clever assumption was to make a clear cut: Langevin proposed that every electron-hole pair with a radius smaller than recombines, all others do not. This is then generalised to account for the carrier concentrations, i.e., these assumptions are used to calculate a recombination current, and from that the recombination rate including the Langevin prefactor.
recombines, all others do not. This is then generalised to account for the carrier concentrations, i.e., these assumptions are used to calculate a recombination current, and from that the recombination rate including the Langevin prefactor.
Your question concerning the last equation is a bit unclear to me. The last equation is , thus the recombination rate is proportional to Langevin recombination strength times electron density times hole density.
, thus the recombination rate is proportional to Langevin recombination strength times electron density times hole density.
Best, C
Tell me if I’m wrong, but I would add that, as far as I understand, there is a very good reason for assuming that the recombination probability is one within the Coulomb radius:
Typically in organic semi-conductors, charges move so slowly that there is very little chances they escape the recombination zone (sphere with $r_c$ radius) before recombination occurs (especially this sphere is so large due to small epsilon).
Exactly the opposite occurs in inorganic crystals: charges are so quick that even they enter the sphere, it is rather unlikely that recombination occurs before they escape it again. That’s even more true for indirect gap semiconductors where the rec rate should be lower I guess, and in any inorganic semiconductor in general because the sphere is much smaller. For this reason Langevin recombination is not even considered in inorganic crystals but rather trap mediated recombination. So recombination where on of the charges is immobile, such as typically Shockley Read Hall recombination.
As far as I understand, in Pope and Swenberg (p.503 or around), they compare the Coulomb radius with the charges mean free path $\lambda$ to set the limit of validity of the “all in sphere recombines and all out escapes recombination” assumption. It works for $\lambda < r_c$.
What I wonder, is the following: given how huge $r_c$ is in organic semiconductors, does it make sense to consider only the attraction potential of 2 charges? Isn't there in average more than this within the sphere?
Actually, the steady state charge carrier concentrations under 1 sun illumination are not so much higher. Setting nm corresponds to a density of just above
nm corresponds to a density of just above  per cubic cm. Also, if there are more than 2 charges within the Coulomb sphere, the calculation does lose its compelling simplicity;-)
per cubic cm. Also, if there are more than 2 charges within the Coulomb sphere, the calculation does lose its compelling simplicity;-)
Thanks, I got it now :)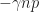 ? Why not
? Why not 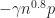 ?
?
Regarding the second question I meant why is it
Not sure where the 0.8 exponent should come from: could you elaborate, please?
Calculating the recombination rate: I realise the recombination current is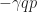 and in addition the recombination rate should also be proportional to electron density. What I don’t understand is why isn’t there a proportionality factor A
and in addition the recombination rate should also be proportional to electron density. What I don’t understand is why isn’t there a proportionality factor A 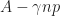
I apologize if I am making this really unclear but I just want to understand :)
Apologies, I meant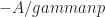
I am really sorry, but I just don’t get your point. Why an exponent of only 0.8 (your previous comment), and where should the proportionality constant come from? Of course, you get a prefactor when solving the continuity equation, but that does not change the recombination rate (which is actually, more precisely,
(which is actually, more precisely, 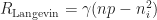 , but that’s another story;-) Experimentally, though, you can of course have deviations from Langevin’s theory; ,in our group, we call this additional prefactor
, but that’s another story;-) Experimentally, though, you can of course have deviations from Langevin’s theory; ,in our group, we call this additional prefactor  .
.
I got it now :) sorry for the confusion. The additional prefactor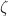 you mentioned clarified it for me. Thanks
you mentioned clarified it for me. Thanks
I am just curious how large those deviations could be? I mean the values of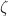
Wow :) didn’t expect it to be that small. That’s so interesting, I’ll keep reading!
Thanks for claryfing those points for me
I jsut didn’t understand what is the Coulomb radius. Can you explain me?
.
I am sorry, there is nothing more to it than written in the post. The particle is free once it does not feel the Coulomb attraction to the other particle any more, which is the case when thermal energy = coulomb energy. That equation is then rearranged to give the Coulomb radius, as described.
Thanks for your useful post. Can you explain the second term n_i^2 in R_Langevin = \gamma *(np-n_i^2)?
I was thinking some holes may have multiple choices (path) to choose which electron to “marry” to, which causes overestimate of the current. Do this term account for this or for other reasons? Thanks.
Thank you very much for your clear explanation.
BTW, do you happen to have an English version of the paper Langevin 1903 (Ann. Chim. Phys. 28, 433)? I want to read the original paper. Thanks.
Unfortunately not, but I recommend you have a look at the book by Pope and Swenberg (1999) for background – or use google translate on Langevin’s original paper;-)
Hey,
I am just trying to understand your paper (phys. rev. b 82, 2010) and I was wondering how you get the connection between what you explain here in this (really nice) blog and the equation R = (q/e0er) (µn+µp)(np-ni^2) labeled with equ. 6 in your paper.
Cheers and greetings to my home area Lower Franconia ;)
Miriam
Hi Miriam, thanks for your comment. In the blog post, the derivation of the Langevin equation is shown. In the paper, we expand the original theory somewhat by explaining the prefactor (which we call ) sometimes necessary to fit experimental polaron dynamics with bimolecular recombination. This prefactor stems in part from spatial gradients of the charge carrier distribution. Thus, if at one end of your device there are many more electrons than holes, the assumption
) sometimes necessary to fit experimental polaron dynamics with bimolecular recombination. This prefactor stems in part from spatial gradients of the charge carrier distribution. Thus, if at one end of your device there are many more electrons than holes, the assumption  (where
(where  is the average carrier concentration which can be determined experimentally) does not work any more. In our paper [Deibel 2010] we just show how the additional prefactor
is the average carrier concentration which can be determined experimentally) does not work any more. In our paper [Deibel 2010] we just show how the additional prefactor  can be calculated if the gradients are known. Does that answer your question? Best wishes, Carsten
can be calculated if the gradients are known. Does that answer your question? Best wishes, Carsten
Hi. Thanks for your great post. It was very useful.
There is only one point unclear to me. As I understand, the calculation is performed under the assumption of zero external electric field (which is of course present in for example OLEDs). How the addition of such a field will affect the recombination? If fact, due to langevin theory any electron that approaches a hole within a distance that Ecoulomb>KT will inevitably recombine. Is this statement true when we have an external electric field?
I would really appreciate if you can help me.
Thanks.
Hi Hadi, thanks for your comment. Indeed Langevin recombination is made for zero field. If you want to extend it, you have to consider that the mobility may be field (and carrier concentration) dependent, and that in principle the Coulomb radius would change. A few years ago I played around with this idea, but have never found a very satisfying answer: it becomes quickly complicated (not even considering “geometry”, charge carrier concentration gradients, and – probably even more important – trapping) the strength of the Langevin theory is that it is so nice and simple, even if not completely correct. Sorry not to be of more help. Best, Carsten
hi.it’s useful post.
why a positive mobile charge move with mobility of electron plus hole?
The positive mobile charge moves only with the hole mobility. This was just to simplify the derivation, which is done for one mobile and one immobile charge. Then, only the mobility of the mobile charge carrier would count. However, considering a case where both charge carriers can move, the mobility in the prefactor becomes the sum of their mobilities.
Hi dear Dr
Please I would juste ask you about the notion of direct and indirect gap in organic semiconductors?
That definition is used for single crystals AFAIK. Crystalline Silicon is an indirect semiconductor and therefore has a low absorption coefficient, whereas amorphous silicon absorbs orders of magnitude better: as good as a direct semiconductor without being one. Indeed, I believe the terms direct / indirect are not really defined for disordered stuff. For organics: Conjugated polymers used for organic photovoltaics are usually rather disordered, have a very high absorption coefficient.
thanks for clarification it’s very kind of you!
I have a question about your second equation. Are you assuming two dimensions when you set the coulombic potential equal to KT? In three dimensions would it be equal to 3/2 KT?
Great post! Thanks for your time.
Hi, thanks! Well, good question. I’d say no in view of my (somewhat) more recent post, https://blog.disorderedmatter.eu/2012/10/16/nongeminate-recombination-langevin-again-and-beyond-later/ m as into which the thermal energy enters cancels out.
into which the thermal energy enters cancels out.
Best, Carsten